Na-Mg-2022-06-12¶

The current structure dataset comprises 38734 structures generated from elemental ICSD structures and binary ICSD prototype alloy structures. A more detailed procedure is found in Phys. Rev. B 102, 174104 (2020).

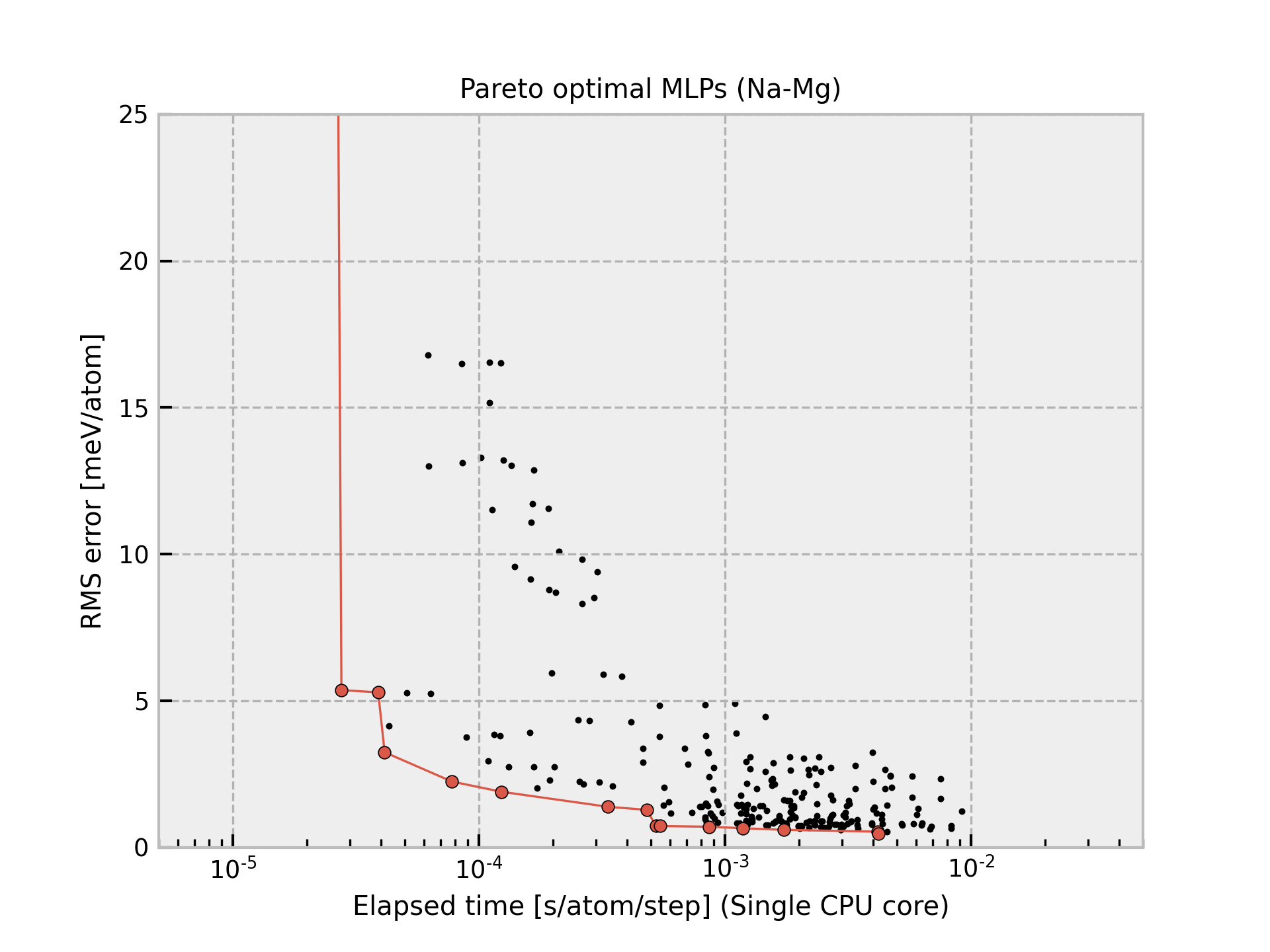
Predictions using Pareto optimal MLPs

../../_images/prediction-ecoh-volume96.png

The cohesive energy and volume are obtained by performing a local structure optimization from the DFT equilibrium structure. In addition, the DFT equilibrium structure is obtained by optimizing a prototype structure included in ICSD, and the prototype is used as the structure legend in the figure. Therefore, the structure type of the converged structure is sometimes different from that shown in the legend even if the potential energy surface predicted by MLP is almost the same as the true one.

The other properties predicted by each Pareto optimal MLP are available from column Predictions in the following table.

Pareto optimals¶
Name	Time [ms] (1core/36cores)	RMSE [meV/atom]/[eV/A]	Predictions	Files
pair-1	0.027 / 0.005	36.320 / 0.0928	–	–
pair-17	0.027 / 0.005	32.397 / 0.0931	–	–
pair-33	0.028 / 0.005	5.3606 / 0.0360	–	–
pair-37	0.039 / 0.005	5.2832 / 0.0343	–	–
pair-49	0.041 / 0.005	3.2407 / 0.0283	predictions	`mlp.lammps` `input` `log`
pair-50	0.078 / 0.008	2.2429 / 0.0207	predictions	`mlp.lammps` `input` `log`
pair-51	0.123 / 0.014	1.8939 / 0.0192	predictions	`mlp.lammps` `input` `log`
pair-60	0.335 / 0.025	1.3797 / 0.0201	predictions	`mlp.lammps` `input` `log`
gtinv-725	0.481 / 0.044	1.2774 / 0.0129	predictions	`mlp.lammps` `input` `log`
gtinv-182	0.525 / 0.035	0.7240 / 0.0137	predictions	`mlp.lammps` `input` `log`
gtinv-254	0.546 / 0.036	0.7240 / 0.0137	predictions	`mlp.lammps` `input` `log`
gtinv-411	0.864 / 0.056	0.7006 / 0.0115	predictions	`mlp.lammps` `input` `log`
gtinv-827	1.181 / 0.079	0.6500 / 0.0086	predictions	`mlp.lammps` `input` `log`
gtinv-591	1.732 / 0.125	0.5954 / 0.0078	predictions	`mlp.lammps` `input` `log`
gtinv-177	4.188 / 0.281	0.5315 / 0.0077	predictions	`mlp.lammps` `input` `log`
gtinv-633	4.199 / 0.297	0.4623 / 0.0075	predictions	`mlp.lammps` `input` `log`

Column “Time” shows the time required to compute the energy and forces for 1 MD step and 1 atom, which is estimated from a simulation of 10 runs for a structure with 284 atoms using a workstation with Intel(R) Xeon(R) CPU E5-2695 v4 @ 2.10GHz. Note that the MLPs should be carefully used for extreme structures. The MLPs often return meaningless values for them.

All Pareto optimal MLPs are available here.